How Not to Fall Off Your Rocker
or
Rocking Chair Radii as Easy as Pi
or
Rocking Chair Radii as Easy as Pi
When Evan Eschmeyer, the smiling, six foot eleven inch NBA player drafted in the second round of the 1999 season by the New Jersey Nets, came to my shop looking for a rocking chair to fit him perfectly; I was prepared! Although I did not know Evan until he walked through the door of my work shop, I had been studying how to make his chair for the past thirteen years. This is the story of my learning to make Evan’s rocking chair.
Since the making of my first rocking chair for my daughter Rachael, sixteen years ago, I have been intent on making every chair I make fit their new owner perfectly. Following the adage that “form should follow function” I believe it is self evident that the function of a rocking chair is to be exquisitely comfortable. If it does not fit properly then it can not be as comfortable as it should be.
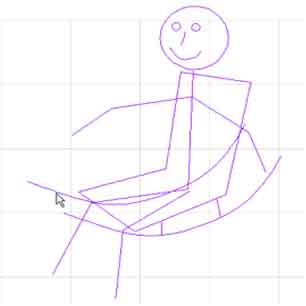
As important as the fit, is the way the rocker rocks. This is determined by the radius of the rockers. As people go beyond average height the radius must change. How much it should change is the question that is addressed here.
When I made my second rocking chair (the first one I intended to sell) I found it did not rock worth a hoot! After much head scratching and gnashing of teeth I decreased the radius of the rockers a good bit and to my surprise it rocked beautifully. Thus, I learned early on, the importance of the rocker radius. I continued making rockers, using the same radius for several years. You know, “If it ain’t broke.” In making rocking chairs fit their new owners perfectly I have changed all dimensions of my rockers many times over. In the process I developed three (I now have six) standard sizes of chairs for folks who order over the internet. It was in the making of a rocking chair for a very long legged fellow that required me to lengthen the legs of my tallest rocker by a full inch and a half that I learned another important lesson about rocker radiuses. When I completed this chair and sat down in it I felt compelled to HANG ON to the arms as the rock was a little on the scary side! I had to increase the radius of the rockers on this chair in order for it to rock properly. The lesson here was that the ideal radius of the rockers appeared to be a function of the leg length. After this experience I began to investigate rocking chairs on the basis of the rocker radius (RR) over the leg length (LL) I have adopted the term RR/LL to express this relationship. The leg length I use for these calculations is the total apparent leg length, the distance from the sitters butt to the floor when the chair seat is parallel to the floor.
I took Calculus in college and don’t remember much of it now but the one thing that stuck with me was the value of looking at minimum and maximum values in order to evaluate functions. The min and max values of a rockers leg length would look something like this:
Since the making of my first rocking chair for my daughter Rachael, sixteen years ago, I have been intent on making every chair I make fit their new owner perfectly. Following the adage that “form should follow function” I believe it is self evident that the function of a rocking chair is to be exquisitely comfortable. If it does not fit properly then it can not be as comfortable as it should be.
As important as the fit, is the way the rocker rocks. This is determined by the radius of the rockers. As people go beyond average height the radius must change. How much it should change is the question that is addressed here.
When I made my second rocking chair (the first one I intended to sell) I found it did not rock worth a hoot! After much head scratching and gnashing of teeth I decreased the radius of the rockers a good bit and to my surprise it rocked beautifully. Thus, I learned early on, the importance of the rocker radius. I continued making rockers, using the same radius for several years. You know, “If it ain’t broke.” In making rocking chairs fit their new owners perfectly I have changed all dimensions of my rockers many times over. In the process I developed three (I now have six) standard sizes of chairs for folks who order over the internet. It was in the making of a rocking chair for a very long legged fellow that required me to lengthen the legs of my tallest rocker by a full inch and a half that I learned another important lesson about rocker radiuses. When I completed this chair and sat down in it I felt compelled to HANG ON to the arms as the rock was a little on the scary side! I had to increase the radius of the rockers on this chair in order for it to rock properly. The lesson here was that the ideal radius of the rockers appeared to be a function of the leg length. After this experience I began to investigate rocking chairs on the basis of the rocker radius (RR) over the leg length (LL) I have adopted the term RR/LL to express this relationship. The leg length I use for these calculations is the total apparent leg length, the distance from the sitters butt to the floor when the chair seat is parallel to the floor.
I took Calculus in college and don’t remember much of it now but the one thing that stuck with me was the value of looking at minimum and maximum values in order to evaluate functions. The min and max values of a rockers leg length would look something like this:
Minimum Leg Length
If you had a rocker with a 42 inch rocker radius and made the legs zero inches tall would it rock well? No, because a forward or backward force applied where the legs would be would simply result in the rocker scooting forward or back, not rocking.
Maximum Leg Length
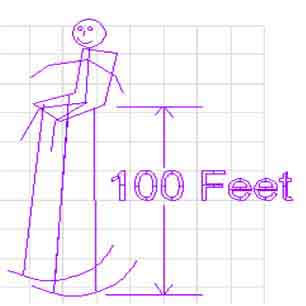
Now, conversely, imagine a rocking chair with a 42 inch rocker radius and legs 100 feet long. Yes, I know 100 feet is extreme but you need big numbers for the min max thing to work, the bigger the better. What will happen to this guy? Well, the lever arm of 100 feet applied to a circle with a 42 inch radius will result in a one way ticket to the ground!
He Will Crash!!
Why is he going to crash? Because the leg length exceeds the radius of the rockers! This would be exactly analogous to a person on a unicycle removing their feet from the pedals. Why is this? Well, if we take a look at circles (what grown up radii are) it becomes a little more clear.
If you apply a weight and a horizontal force towards the bottom of a circle it will be fairly stable and resistant to moving. This RR/LL would be very high, 10 to 20. This would be like trying to move a bicycle wheel by pushing on the valve stem when it is on the bottom. It will move a little then ‘scoot’, exactly like a rocking chair with too large a rocker radius.
Now, apply a weight and a horizontal force at the center of a circle. The circle will be free to roll where ever it likes. Once an individual started to rock (by applying a horizontal force) the circle would keep rolling in what ever direction the initial force was applied because the weight, in this case, does not influence the position of the circle. This would not be ideal for a rocking chair. This situation represents an RR/LL of ONE. Clearly too small for a stable rock. Again, if you applied force at the axle of a bicycle wheel, it would continue to roll until it ran into something.
So far, I believe we can intuit that a RR/LL of one (weight in the center) will not work and also that a VERY small RR/LL would not be suitable either, so the answer must be somewhere in between. I believe it is safe to assume that all circles behave the same. Therefore I will also assume that there is only one RR/LL that provides an ideal oscillation back and forth, always returning to center and never over rotating, that is perfect for rocking. (Disregarding personal taste for the moment) No matter how large or small the circle, the ratio will remain constant. I also conclude that the function is clearly of a linear nature. So – What is this magical function and can it really be simple and linear? I began to wonder if this number might not be one already known to everyone and famous for some purpose other than rocking chairs. The Golden Mean came to mind. I started to do some testing. As stated earlier, the point at which the sitter’s back side impinges upon the top of the seat is taken as the leg length for the following calculations. I measured all three of my standard rockers, Large, Medium and Small and using a rocker radius of 42” (my standard radius), here is what I came up with.
- Large Leg Length = 14.33 RR/LL = 2.93
- Medium Leg Length = 13.42 RR/LL = 3.13
- Small Leg Length = 12.5 RR/LL = 3.36
Two point ninety three to three point thirty six is fairly close. Every size rocking chair, I would think, should have a specific rocker radius. If I am using the same radius rockers on three sizes of chairs then any one of them is perfect, the other two must be off a little. Taking the average of all three sizes results in a number strikingly close to Pi. I find that fascinating! Wouldn’t you know it would be a number that every rocking chair maker knows about but didn’t associate with rocker radii.
During this same time frame I was commissioned to make a chair for a Major in the Air Force, David Hoopes, that was six foot seven inches tall AND long legged! He required a rocker with a seat that was an inch and a half taller than my current largest rocking chair. I knew this chair was going to require a new rocker form in order to rock properly from my work thus far. So what radius should I use? With a projected new seat height of 15.8 inches, multiplying that by 3 (I didn’t use 3.14 at this time because I had not made the connection to Pi) resulted in a new radius of very close to 47 inches. I made the form with a 44 inch radius, expecting the usual spring back after removal from the gluing form to result in a radius very close to a 47 inches. It worked perfectly!
During this same time frame I was commissioned to make a chair for a Major in the Air Force, David Hoopes, that was six foot seven inches tall AND long legged! He required a rocker with a seat that was an inch and a half taller than my current largest rocking chair. I knew this chair was going to require a new rocker form in order to rock properly from my work thus far. So what radius should I use? With a projected new seat height of 15.8 inches, multiplying that by 3 (I didn’t use 3.14 at this time because I had not made the connection to Pi) resulted in a new radius of very close to 47 inches. I made the form with a 44 inch radius, expecting the usual spring back after removal from the gluing form to result in a radius very close to a 47 inches. It worked perfectly!
David Hoopes and his new rocking chair
Combining the data on all of the chairs I make, the data looks pretty interesting.
|
Size
Extra Large Large Medium Small Child's |
Seat Height
15.87 14.33 13.42 12.5 10.74 |
RR/LL
2.96 2.9 3.12 3.36 2.9 |
I would venture to say that anybody who would like a “good rocking” rocking chair should have a RR/LL ratio from 2.9 to 3.2. (How about using Pi!) By the way, the guy on the 100 foot tall rocker would have lived if his rocker radius had been 314 feet!
So, what should I do with Evan’s rocker radius? He required a seat one inch taller than David Hoop’s chair (and a headrest two full inches higher). Therefore, 16.87 times Pi equals 53 inches. Again, I made the form smaller to compensate for spring back. Therefore Evans rocker form was made at a 48 inch radius, Four inches larger than the one for David. The result was a rocker with a perfect rocking action.
So, what should I do with Evan’s rocker radius? He required a seat one inch taller than David Hoop’s chair (and a headrest two full inches higher). Therefore, 16.87 times Pi equals 53 inches. Again, I made the form smaller to compensate for spring back. Therefore Evans rocker form was made at a 48 inch radius, Four inches larger than the one for David. The result was a rocker with a perfect rocking action.
Evan Eschmeyer - with his sneakers on is 7 feet tall
What about Center of gravity?
It is my opinion that RR/LL trumps COG calculations in a rocking chair. Why? Well, in the beginning I thought it was odd that people of ALL sizes could sit in my small rocker and exclaim that it rocked beautifully and was the most comfortable chair they ever sat in. Did the COG change with each person? Absolutely! Did it make any difference to the comfort or the quality of the rock? It didn’t seem so to me! Each individual would, of course, be more comfortable in a rocking chair that fit them perfectly but it was the RR/LL that seemed to be the most important factor.
There is a very good reason for this. It is because the rock back in a rocking chair is governed by the length of the lever arm used to propel the rocker backwards in it’s arc. The ‘lever arm’ is the LL used in my formula RR/LL = 3.14. No matter how tall or short the person sitting in the rocker the ‘lever arm’ remains constant. Therefore the rock would be the same for everyone.
Now, it would appear that the return portion of the rock is governed by the old “COG” I believe it is a bigger factor on the return trip but as it is moderated by the sitter’s foot pressure and movement, “COG does not have exclusive control even on the return trip.
There is a very good reason for this. It is because the rock back in a rocking chair is governed by the length of the lever arm used to propel the rocker backwards in it’s arc. The ‘lever arm’ is the LL used in my formula RR/LL = 3.14. No matter how tall or short the person sitting in the rocker the ‘lever arm’ remains constant. Therefore the rock would be the same for everyone.
Now, it would appear that the return portion of the rock is governed by the old “COG” I believe it is a bigger factor on the return trip but as it is moderated by the sitter’s foot pressure and movement, “COG does not have exclusive control even on the return trip.
Empirical Evidence
If I increase the length of the legs on my large rocker by a full two inches the rock will be completely out of control. Way too much action back and forth, a good bit on the scary side. I assure you NO ONE (except a 12 year old) would enjoy the rock!
What has happened? I have lengthened the "lever arm" and also changed the COG by two inches. Which one effects the change in rocker action? If someone five feet tall sits in my large rocker they will think it rocks well. Another person six feet four inches can sit in the SAME CHAIR and exclaim that it rocks beautifully. Has the COG changed more than two inches, caused by the extreme difference in the height of the sitter?? Absolutely! Did it affect the rock? Absolutely NOT it stayed the same! Did the "lever arm" (leg length) change? Absolutely NOT.
It looked to me like I had figured it out. Now, did I have the courage to put my theory to the test? Not having the time or space to make a 100 foot rocking chair I settled on a six foot tall version. That is six feet from the top of the seat to the ground.
Six feet times 3.14 results in a theoretical rocker radius of 19 feet. I got my big tape out and scribed a 19 foot radius on a couple of one bys and constructed my rocker.
What has happened? I have lengthened the "lever arm" and also changed the COG by two inches. Which one effects the change in rocker action? If someone five feet tall sits in my large rocker they will think it rocks well. Another person six feet four inches can sit in the SAME CHAIR and exclaim that it rocks beautifully. Has the COG changed more than two inches, caused by the extreme difference in the height of the sitter?? Absolutely! Did it affect the rock? Absolutely NOT it stayed the same! Did the "lever arm" (leg length) change? Absolutely NOT.
It looked to me like I had figured it out. Now, did I have the courage to put my theory to the test? Not having the time or space to make a 100 foot rocking chair I settled on a six foot tall version. That is six feet from the top of the seat to the ground.
Six feet times 3.14 results in a theoretical rocker radius of 19 feet. I got my big tape out and scribed a 19 foot radius on a couple of one bys and constructed my rocker.
Getting on was not straight forward.
Finally on!
Rocking back.
Rocking forward!
It rocked perfectly, giving me a very secure and pleasurable traverse through the upper atmosphere.
It is clear to me that anyone making any type of rocking device could use Pi to calculate the perfect rocker shape and save themselves a lot of experimentation.
Finally on!
Rocking back.
Rocking forward!
It rocked perfectly, giving me a very secure and pleasurable traverse through the upper atmosphere.
It is clear to me that anyone making any type of rocking device could use Pi to calculate the perfect rocker shape and save themselves a lot of experimentation.